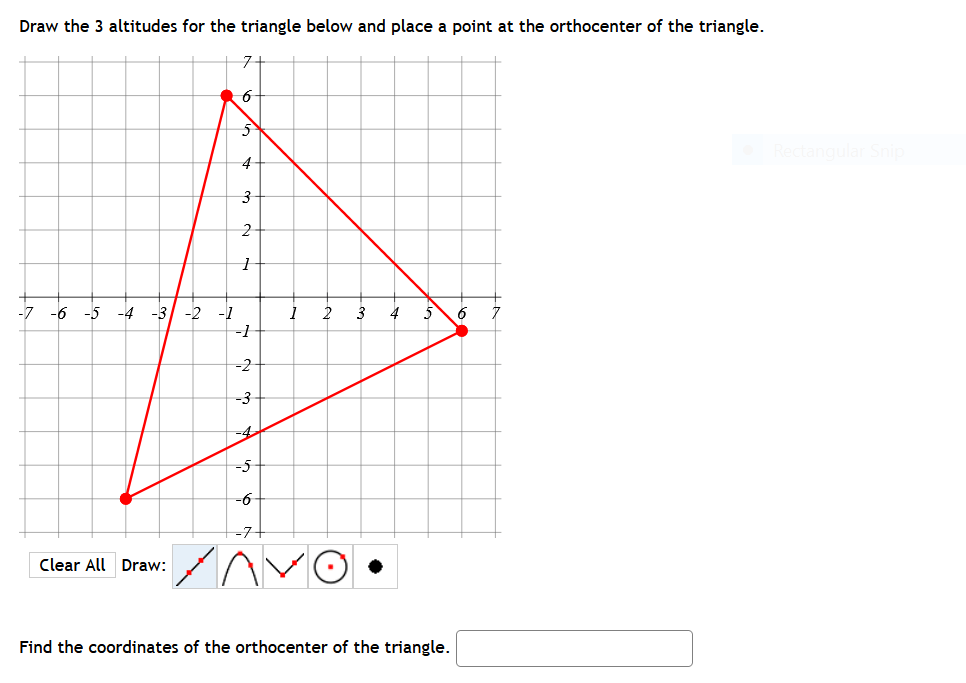
How To Draw The Orthocenter Of A Triangle
How To Draw The Orthocenter Of A Triangle - The orthocenter is the point where all three altitudes of the triangle intersect. So we know (r, r). Web the main three main aspects of an orthocenter are: This is because the orthocenter is the intersection of the altitudes, which are. All the perpendiculars drawn from. To start, let's assume that the triangle abc. Web what is the orthocenter of a triangle? Draw the triangle and label the vertices as a, b, and c. For a more, see orthocenter of a triangle. Define the orthocenter and learn how to find the orthocenter of a triangle in four steps with this free geometry video.
💡 find the coordinates of the. See constructing the the orthocenter of a triangle. Construct an altitude from a vertex of the triangle to the opposite side, or the line containing the opposite side. Web orthocenter of a triangle is the point of intersection of all the perpendiculars to the sides of the triangle drawn from each vertex. All the perpendiculars drawn from. Where the three perpendicular bisectors of the sides of a triangle intersect (a perpendicular bisector is a line that forms a 90° angle with a. We have seen how to construct an altitude of a triangle.
Web there are some remarkable facts. While an acute triangle 's orthocenter is located in the. Web this page shows how to construct the orthocenter of a triangle with compass and straightedge or ruler. Web steps for constructing the orthocenter of a triangle. Web see constructing the orthocenter of a triangle.
Orthocenter Of A Triangle
DRAW AN ACUTE ANGLE TRIANGLE . DRAW ALL ITS ALTITUDE AND SHOW THE
Solved Draw the 3 altitudes for the triangle below and place
How to draw Orthocenter of a Triangle YouTube
How to Draw Altitudes of a Triangle & Orthocenter YouTube
How To Draw An Obtuse Triangle at How To Draw
What is the orthocentre of a triangle?
Define the orthocenter and learn how to find the orthocenter of a triangle in four steps with this free geometry video. By calling n the midpoint of oh we have that. Simply construct the three altitudes of the triangle. Where the three perpendicular bisectors of the sides of a triangle intersect (a perpendicular bisector is a line that forms a 90° angle with a. An altitude is a line segment drawn from a vertex of the triangle perpendicular to the opposite. See constructing the the orthocenter of a triangle.
If the orthocenter and centroid are the same point, then the triangle is equilateral. So we know (r, r). Web this page shows how to construct the orthocenter of a triangle with compass and straightedge or ruler. By calling n the midpoint of oh we have that. Web orthocenter of a triangle is the point of intersection of all the perpendiculars to the sides of the triangle drawn from each vertex.
We have seen how to construct an altitude of a triangle. An altitude is a line segment drawn from a vertex of the triangle perpendicular to the opposite. The illustration above demonstrates that the orthocenter of an obtuse triangle is situated in the triangle's exterior; By calling n the midpoint of oh we have that.
Web Learn How To Use A Compass And A Straightedge To Construct The Orthocenter Of A Triangle!
The orthocenter is the point where all three altitudes of the triangle intersect. Web the main three main aspects of an orthocenter are: See constructing the the orthocenter of a triangle. So we know (r, r).
This Is Because The Orthocenter Is The Intersection Of The Altitudes, Which Are.
While an acute triangle 's orthocenter is located in the. Web orthocenter of a triangle is the point of intersection of all the perpendiculars to the sides of the triangle drawn from each vertex. By calling n the midpoint of oh we have that. Web this video shows how to construct the orthocenter of a triangle by constructing altitudes of the triangle.
All The Perpendiculars Drawn From.
If the orthocenter and centroid are the same point, then the triangle is equilateral. Construct the perpendicular bisectors of the sides. Use this information to help you in your geometry class! Define the orthocenter and learn how to find the orthocenter of a triangle in four steps with this free geometry video.
Web There Are Some Remarkable Facts.
The construction uses only a compass and straight. The construction starts by extending the chosen side of the triangle in both directions. Web the orthocenter of a triangle is the point where the altitudes of the triangle intersect. For a more, see orthocenter of a triangle.